3.Station: Unterschied zwischen den Versionen
Aus DMUW-Wiki
(Formel bearbeitet) |
(Herleitung verbessert) |
||
| Zeile 6: | Zeile 6: | ||
==3. Station: Berechnung der Streckenlängen und des Streckungsfaktors== | ==3. Station: Berechnung der Streckenlängen und des Streckungsfaktors== | ||
:Wie du in der 2. Station schon herausgefunden hast ist die Bildstrecke |k|-mal so lang wie die Urbildstrecke. | :Wie du in der 2. Station schon herausgefunden hast ist die Bildstrecke |k|-mal so lang wie die Urbildstrecke. | ||
| − | :Geometrisch bedeutet dies für einen beliebigen Punkt P: <span style="text-decoration: overline;">ZP'</span> = |k| ∙ <span style="text-decoration: overline;">ZP</span> | + | :Geometrisch bedeutet dies für einen beliebigen Punkt P: '''<span style="color:#00cd00"><span style="text-decoration: overline;">ZP'</span> = |k| ∙ <span style="text-decoration: overline;">ZP</span></span>''' |
:Daraus folgt: <math>\mid k\mid = {\overline{ZP'}\over\overline{ZP}}</math> | :Daraus folgt: <math>\mid k\mid = {\overline{ZP'}\over\overline{ZP}}</math> | ||
<br> | <br> | ||
| Zeile 15: | Zeile 15: | ||
|[[Bild:Porzelt_Streckenlänge.jpg]]|| | |[[Bild:Porzelt_Streckenlänge.jpg]]|| | ||
<div class="lueckentext-quiz"> | <div class="lueckentext-quiz"> | ||
| − | <span style="text-decoration: overline;"> | + | <span style="text-decoration: overline;">ZP'</span> = |k| ∙ <span style="text-decoration: overline;">ZP</span> <math>\wedge</math> <span style="text-decoration: overline;">ZQ'</span> = |k| ∙ <span style="text-decoration: overline;">ZQ</span><br> |
| − | <math>\ | + | <span style="text-decoration: overline;">PQ</span> = <span style="text-decoration: overline;">ZQ</span> - <span style="text-decoration: overline;">ZP</span> <math>\wedge</math> <span style="text-decoration: overline;">P'Q'</span> = <span style="text-decoration: overline;">ZQ'</span> - <span style="text-decoration: overline;">ZP'</span><br> |
| − | <math>\ | + | <math>\Rightarrow </math><span style="text-decoration: overline;">P'Q'</span> = '''|k|''' ∙ <span style="text-decoration: overline;">ZQ</span> - |k| ∙ '''<span style="text-decoration: overline;">ZP</span>'''<br> |
| − | <math>\ | + | <math>\Rightarrow </math><span style="text-decoration: overline;">P'Q'</span> = |k| ∙ ('''<span style="text-decoration: overline;">ZQ</span>''' - '''<span style="text-decoration: overline;">ZP</span>''')<br> |
| + | <math>\Rightarrow </math><span style="text-decoration: overline;">P'Q'</span> = |k| ∙ '''<span style="text-decoration: overline;">PQ</span>''' | ||
</div> | </div> | ||
|} | |} | ||
Version vom 8. Juli 2009, 16:50 Uhr
1. Station: Ähnlichkeitsabbildung - Exkurs: Weitere Beispiele einer zentrischen Streckung - 2. Station: Streckungsfaktor - Fortsetzung der 2. Station: Streckungsfaktor - 3. Station: Berechnung der Streckenlängen und des Streckungsfaktors - 4. Station: Zusammenfassung - 5. Station: Übungen - 6. Station: Wissenswertes
3. Station: Berechnung der Streckenlängen und des Streckungsfaktors
- Wie du in der 2. Station schon herausgefunden hast ist die Bildstrecke |k|-mal so lang wie die Urbildstrecke.
- Geometrisch bedeutet dies für einen beliebigen Punkt P: ZP' = |k| ∙ ZP
- Daraus folgt:
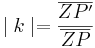
- Ob dies auch zur Berechnung von Strecken, die nicht durch den Punkt Z verlaufen, gilt, kannst du durch Umformung herausfinden.
- Setze dafür den richtigen Ausdruck in die passende Lücke:
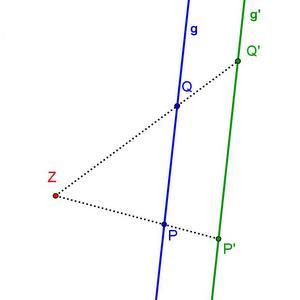 |
ZP' = |k| ∙ ZP ZPZPZQPQ|k| |