2.Station: Unterschied zwischen den Versionen

Aus DMUW-Wiki
Wechseln zu: Navigation, Suche
K
 
(19 dazwischenliegende Versionen von 2 Benutzern werden nicht angezeigt)
Zeile 1: Zeile 1:
 
<div style="margin:0; margin-right:4px; margin-left:0px; border:2px solid #f4f0e4; padding: 0em 0em 0em 1em; background-color:#f4f0e4;">
 
<div style="margin:0; margin-right:4px; margin-left:0px; border:2px solid #f4f0e4; padding: 0em 0em 0em 1em; background-color:#f4f0e4;">
−
[[Benutzer:Leonie Porzelt/Vierstreckensatz|1. Station: Erster Vierstreckensatz - Schenkellösung]] - [[Benutzer:Leonie Porzelt/Vierstreckensatz/2.Station|2. Station: Erster Vierstreckensatz - Abschnittlösung]] - [[Benutzer:Leonie Porzelt/Vierstreckensatz/3.Station|3. Station: Zweiter Vierstreckensatz]] - [[Benutzer:Leonie Porzelt/Vierstreckensatz/4.Station|4. Station: Zusammenfassung]] - [[Benutzer:Leonie Porzelt/Vierstreckensatz/5.Station|5. Station: Übung]]
+
[[Lernpfade/Zentrische Streckung/Vierstreckensatz|1. Station: Erster Vierstreckensatz - Schenkellösung]] - [[Lernpfade/Zentrische Streckung/Vierstreckensatz/2.Station|2. Station: Erster Vierstreckensatz - Abschnittlösung]] - [[Lernpfade/Zentrische Streckung/Vierstreckensatz/3.Station|3. Station: Zweiter Vierstreckensatz]] - [[Lernpfade/Zentrische Streckung/Vierstreckensatz/4.Station|4. Station: Zusammenfassung]] - [[Lernpfade/Zentrische Streckung/Vierstreckensatz/5.Station|5. Station: Übung]]
 
</div>
 
</div>
 
<br>
 
<br>
  
 
==2. Station: Erster Vierstreckensatz - Abschnittlösung==
 
==2. Station: Erster Vierstreckensatz - Abschnittlösung==
−
:Bei dieser Aufgabe ist die gesuchte Größe nur ein Abschnitt des Schenkels.
+
Bei dieser Aufgabe sollst du berechnen, wie weit Dia von Panto entfernt ist. Die gesuchte Größe ist hier nur ein<br>
−
[[Bild:Porzelt_Vierstreckensatz_Abschnittlösung.jpg]]
+
Abschnitt des Schenkels.<br>
−
[[Bild:Porzelt_Idee.jpg]]
+
[[Bild:Porzelt_Idee.jpg]]<br>
−
:Anhand der Eigenschaft der Längenverhältnisstreue der zentrischen Streckung, kannst du auch hier wieder die geeignete Formel
+
Anhand der Eigenschaft der Längenverhältnisstreue der zentrischen Streckung kannst du auch hier wieder die geeignete Formel<br>
−
:zur Berechnung der unbekannten Strecke herleiten. Setze dafür die richtige Aussage in die passende Lücke ein:
+
zur Berechnung der unbekannten Strecke herleiten. Setze dafür die richtige Aussage in die passende Lücke ein:<br>
 +
<div style="border: 2px solid #0000ff; background-color:#ffffff; padding:7px;">
 
<div class="lueckentext-quiz">
 
<div class="lueckentext-quiz">
−
<math>\overline{AA'}</math> = '''|k| ∙ <math>\overline{ZA}</math> - <math>\overline{ZA}</math>''' <math>\wedge</math> <math>\overline{BB'}</math> = '''|k| ∙ <math>\overline{ZB}</math> - <math>\overline{ZB}</math>'''<br>
+
<math>\overline{ZA'} =</math> '''<math>|k| \cdot \overline{ZA}</math>''' <math>\mathit{und}\ \overline{ZB'} =</math> '''<math>|k| \cdot \overline{ZB}</math>'''<br>
 +
<math>\overline{AA'} = \overline{ZA'} - \overline{ZA}\ \mathit{und}\ \overline{BB'} = \overline{ZB'} - \overline{ZB}</math><br>
 +
Erste Zeile in zweite Zeile eingesetzt ergibt:<br>
 +
<math>\overline{AA'} =</math> '''<math>|k| \cdot \overline{ZA} - \overline{ZA}</math>''' <math>\mathit{und}\ \overline{BB'} =</math> '''<math>|k| \cdot \overline{ZB} - \overline{ZB}</math>'''<br>
 
Aufgelöst nach |k|:<br>
 
Aufgelöst nach |k|:<br>
−
|k| = '''<math>{\overline{AA'}\over\overline{ZA}}</math>''' - <math>{\overline{ZA}\over\overline{ZA}}</math> <math>\wedge</math> |k| = <math>{\overline{BB'}\over\overline{ZB}}</math> - '''<math>{\overline{ZB}\over\overline{ZB}}</math>'''<br>
+
<math>\mid k\mid =</math> '''<math>{\overline{AA'}\over\overline{ZA}}</math>''' <math>- {\overline{ZA}\over\overline{ZA}}</math> <math>\mathit{und}\ \mid k\mid =</math> <math>{\overline{BB'}\over\overline{ZB}} - </math>'''<math>{\overline{ZB}\over\overline{ZB}}</math>'''<br>
−
|k| = '''<math>{\overline{AA'}\over\overline{ZA}}</math> - 1''' <math>\wedge</math> '''|k|''' = <math>{\overline{BB'}\over\overline{ZB}}</math> - 1<br>
+
<math>\mid k\mid =</math> '''<math>{\overline{AA'}\over\overline{ZA}} - 1</math>''' <math>\mathit{und}\ </math> '''<math>\mid k\mid </math>''' <math>= {\overline{BB'}\over\overline{ZB}} - 1</math><br>
 
Gleichsetzen:<br>
 
Gleichsetzen:<br>
−
<math>{\overline{AA'}\over\overline{ZA}}</math> - '''1''' = '''<math>{\overline{BB'}\over\overline{ZB}}</math> '''- 1 '''|+1'''<br>
+
<math>{\overline{AA'}\over\overline{ZA}} - 1 =</math> '''<math>{\overline{BB'}\over\overline{ZB}}</math> '''<math>- 1 \mid+1</math><br>
−
<math>{\overline{AA'}\over\overline{ZA}}</math> = <math>{\overline{BB'}\over\overline{ZB}}</math>
+
<math>{\overline{AA'}\over\overline{ZA}} =</math> <math>{\overline{BB'}\over\overline{ZB}}</math>
 +
</div>
 +
&nbsp;
 
</div>
 
</div>
 
<br>
 
<br>
−
:Super! Du hast die '''Abschnittlösung des ersten Vierstreckensatzes''' hergeleitet.  
+
<div style="border: 2px solid #cfcfcf; background-color:#ffffff; padding:7px;">
 +
{|
 +
|Du hast die '''Abschnittlösung des ersten Vierstreckensatzes''' hergeleitet.||&nbsp;
 +
[[Bild:Porzelt_lobenderPanto9.jpg]]
 +
|}
 +
</div>
 +
<br>
 +
 
 
<div style="border: 2px solid #ffd700; background-color:#ffffff; padding:7px;">
 
<div style="border: 2px solid #ffd700; background-color:#ffffff; padding:7px;">
 
[[Bild:Porzelt_Panto-2.jpg|left]]
 
[[Bild:Porzelt_Panto-2.jpg|left]]
 
<br>
 
<br>
−
:Auch hier verhalten sich die Abschnitte auf der einen Halbgeraden, wie die Abschnitte auf der anderen Halbgeraden.
+
Auch hier verhalten sich die Abschnitte auf der einen Halbgeraden wie die Abschnitte auf der anderen Halbgeraden.<br>
 
<br>
 
<br>
 
</div>
 
</div>
 
<br>
 
<br>
−
:Berechne nun die Aufgabe in deinem Heft und trage hier deine Lösung mit Angabe der Einheit (cm) ein!
+
'''Trage hier deine Lösung mit Angabe der Einheit (cm) ein!'''<br>
 +
<div style="border: 2px solid #00cd00; background-color:#ffffff; padding:7px;">
 
<div class="lueckentext-quiz">
 
<div class="lueckentext-quiz">
−
x = '''1 cm (Tipp:  Leerzeichen zwischen Zahl und Einheit nicht vergessen!)'''.
+
<math>{x \over 2\ cm} = {5\ cm \over 2,5\ cm}</math><br>
 +
Berechne das Ergebnis mit dem Taschenrechner:<br>
 +
x = '''4 cm (Tipp:  Leerzeichen zwischen Zahl und Einheit nicht vergessen!)'''.
 
</div>
 
</div>
 +
&nbsp;
 +
</div>
 +
<br>
 +
<div align="left">[[Lernpfade/Zentrische Streckung/Vierstreckensatz/3.Station|<math>\Rightarrow</math> Weiter zur 3. Station: Zweiter Vierstreckensatz]]</div>
 
<br>
 
<br>
−
<div align="right">[[Benutzer:Leonie Porzelt/Vierstreckensatz/3.Station|Weiter zur 3. Station: Zweiter Vierstreckensatz]]</div>
+
<div align="left">[[Lernpfade/Zentrische Streckung/Vierstreckensatz|<math>\Leftarrow</math> Zurück zur 1. Station: Erster Vierstreckensatz - Schenkellösung]]</div>
−
<div align="left">[[Benutzer:Leonie Porzelt/Vierstreckensatz|Zurück zur 1. Station: Erster Vierstreckensatz - Schenkellösung]]</div>
+

Aktuelle Version vom 13. Juli 2009, 14:22 Uhr

1. Station: Erster Vierstreckensatz - Schenkellösung - 2. Station: Erster Vierstreckensatz - Abschnittlösung - 3. Station: Zweiter Vierstreckensatz - 4. Station: Zusammenfassung - 5. Station: Übung


2. Station: Erster Vierstreckensatz - Abschnittlösung

Bei dieser Aufgabe sollst du berechnen, wie weit Dia von Panto entfernt ist. Die gesuchte Größe ist hier nur ein
Abschnitt des Schenkels.
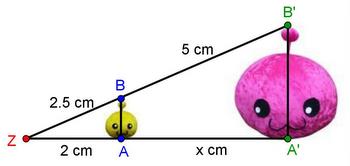
Porzelt Idee.jpg
Anhand der Eigenschaft der Längenverhältnisstreue der zentrischen Streckung kannst du auch hier wieder die geeignete Formel
zur Berechnung der unbekannten Strecke herleiten. Setze dafür die richtige Aussage in die passende Lücke ein:

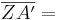
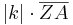
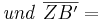
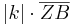
\overline{ZA'} = |k| \cdot \overline{ZA} \mathit{und}\ \overline{ZB'} = |k| \cdot \overline{ZB}
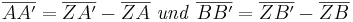
\overline{AA'} = \overline{ZA'} - \overline{ZA}\ \mathit{und}\ \overline{BB'} = \overline{ZB'} - \overline{ZB}
Erste Zeile in zweite Zeile eingesetzt ergibt:
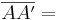
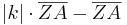
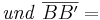
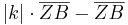
\overline{AA'} = |k| \cdot \overline{ZA} - \overline{ZA} \mathit{und}\ \overline{BB'} = |k| \cdot \overline{ZB} - \overline{ZB}
Aufgelöst nach |k|:
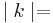
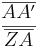
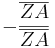
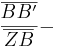
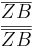
\mid k\mid = {\overline{AA'}\over\overline{ZA}} - {\overline{ZA}\over\overline{ZA}} \mathit{und}\ \mid k\mid = {\overline{BB'}\over\overline{ZB}} - {\overline{ZB}\over\overline{ZB}}
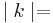
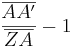
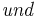
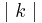
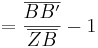
\mid k\mid = {\overline{AA'}\over\overline{ZA}} - 1 \mathit{und}\ \mid k\mid = {\overline{BB'}\over\overline{ZB}} - 1
Gleichsetzen:
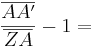
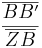
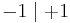
{\overline{AA'}\over\overline{ZA}} - 1 = {\overline{BB'}\over\overline{ZB}} - 1 \mid+1
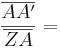
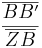
{\overline{AA'}\over\overline{ZA}} = {\overline{BB'}\over\overline{ZB}}

 


Du hast die Abschnittlösung des ersten Vierstreckensatzes hergeleitet.  

Porzelt lobenderPanto9.jpg


Porzelt Panto-2.jpg


Auch hier verhalten sich die Abschnitte auf der einen Halbgeraden wie die Abschnitte auf der anderen Halbgeraden.


Trage hier deine Lösung mit Angabe der Einheit (cm) ein!

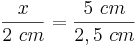
{x \over 2\ cm} = {5\ cm \over 2,5\ cm}
Berechne das Ergebnis mit dem Taschenrechner:
x = 4 cm (Tipp: Leerzeichen zwischen Zahl und Einheit nicht vergessen!).

 


\Rightarrow Weiter zur 3. Station: Zweiter Vierstreckensatz


\Leftarrow Zurück zur 1. Station: Erster Vierstreckensatz - Schenkellösung
Von „http://dmuw.zum.de/index.php?title=Lernpfade/Zentrische_Streckung/Vierstreckensatz/2.Station&oldid=3118“