Algebra: Gleichungen: Unterschied zwischen den Versionen
Aus DMUW-Wiki
K (Die Seite wurde neu angelegt: <span style="background:#CD96CD ">'''Deine neue Lieblings-Merkregel zum Umformen von Termen und Gleichungen:'''</span><br> <div class="schuettel-quiz"> Rechne immer '''...) |
K |
||
| (17 dazwischenliegende Versionen von 2 Benutzern werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| − | < | + | <div style="border: 3px solid #551A8B ; background-color:#CD96CD ; padding:7px;"><big><span style="color:#551A8B ">'''Gleichungen</span>'''</big></div> |
| − | + | <br /> | |
| − | + | ||
| − | </ | + | |
{| | {| | ||
|- | |- | ||
| width="550" | | | width="550" | | ||
| − | '''Wie bei einer Waage müssen beide Seiten der Gleichung immer im Gleichgewicht sein. Dies erreichst du durch | + | <div style="border: 4px solid #551A8B ; background-color:#CD96CD ; padding:7px;"> |
| + | '''Wie bei einer Waage müssen beide Seiten der Gleichung immer im Gleichgewicht sein. Dies erreichst du durch <span style="color:#551A8B ">Äquivalenzumformungen</span>, mit denen du beide Seiten der Gleichung einheitlich umstellst, ohne dass sich der Wert der Gleichung ändert.''' | ||
| + | </div> | ||
|| | || | ||
::[[Bild:Haas_Balkenwaage.jpg|250px]] | ::[[Bild:Haas_Balkenwaage.jpg|250px]] | ||
|} | |} | ||
| − | + | <span style="background:#CD96CD ">'''Deine neue Lieblings-Merkregel zum Umformen von Termen und Gleichungen:'''</span><br> | |
| + | <div class="schuettel-quiz"> | ||
| + | Rechne immer '''Klammer''' vor '''Punkt''' vor '''Strich'''! | ||
| + | </div> | ||
<span style="background:#CD96CD ">'''Wende diese Regel nun auf die folgenden Aufgaben an. Beachte immer die Grundmenge, die rechts steht.'''</span> | <span style="background:#CD96CD ">'''Wende diese Regel nun auf die folgenden Aufgaben an. Beachte immer die Grundmenge, die rechts steht.'''</span> | ||
| Zeile 19: | Zeile 22: | ||
''Erst ganz am Ende kannst du dann dein Ergebnis durch Klick auf "Lösung anzeigen" überprüfen.'' | ''Erst ganz am Ende kannst du dann dein Ergebnis durch Klick auf "Lösung anzeigen" überprüfen.'' | ||
| + | <div style="border: 4px solid #551A8B ; background-color:#00000 ; padding:7px;"> | ||
| + | * <span style="color: #551A8B">'''Beispiel :'''</span> | ||
| + | :<math> \ 9x - 12 = 60</math> | ||
| + | <div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | ||
| + | :<math> \ 9x - 12 = 60 \;\;\;\;\;\;\;\;| + 12 </math> | ||
| − | + | :<math>\Leftrightarrow 9x = 72 \;\;\;\;\;\;\;\;| : 9 </math> | |
| − | <div align="right"><math>\mathbb{G}=\mathbb{ | + | |
| + | :<math>\Leftrightarrow x = 8 </math> | ||
| + | |||
| + | :<math>\Rightarrow\mathbb{L}= \mathcal{f}8\mathcal{g}</math> | ||
| + | </div> | ||
| + | |||
| + | |||
| + | * 3x + 10 = 19 | ||
| + | <div align="right"><math>\mathbb{G}=\mathbb{N}</math></div> | ||
| + | |||
| + | :3x + 10 = 19 | <u style="color:lightgrey;background:lightgrey">- 10 </u> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">3x = 9</u> | <u style="color:lightgrey;background:lightgrey">: 3</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">3x = 9</u> | <u style="color:lightgrey;background:lightgrey">: 3</u> |
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 3</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 3</u> | ||
| Zeile 31: | Zeile 49: | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
| − | ::<math> \ 3x + 10 = 19 | - 10 </math> | + | ::<math> \ 3x + 10 = 19 \;\;\;\;\;\;\;\;| - 10 </math> |
| − | ::<math>\Leftrightarrow 3x = 9 | : 3 </math> | + | ::<math>\Leftrightarrow 3x = 9 \;\;\;\;\;\;\;\;| : 3 </math> |
::<math>\Leftrightarrow x = 3 </math> | ::<math>\Leftrightarrow x = 3 </math> | ||
| Zeile 40: | Zeile 58: | ||
}} | }} | ||
| − | * 9x - 18 = 27 | + | * 9x - 18 = 27 |
<div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | <div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">9x = 45</u> | <u style="color:lightgrey;background:lightgrey">: 9</u> | + | :9x - 18 = 27 | <u style="color:lightgrey;background:lightgrey"> + 18 </u> |
| + | |||
| + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">9x = 45</u> | <u style="color:lightgrey;background:lightgrey">: 9</u> | ||
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 5</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 5</u> | ||
| Zeile 50: | Zeile 70: | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
| − | ::<math> \ 9x - 18 = 27 | + 18 </math> | + | ::<math> \ 9x - 18 = 27 \;\;\;\;\;\;\;\;| + 18 </math> |
| − | ::<math>\Leftrightarrow 9x = | + | ::<math>\Leftrightarrow 9x = \;\;\;\;\;\;\;\;| : 9 </math> |
::<math>\Leftrightarrow x = 5 </math> | ::<math>\Leftrightarrow x = 5 </math> | ||
| Zeile 63: | Zeile 83: | ||
<div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | <div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 12 - 1 = 2x + 1</u> | <u style="color:lightgrey;background:lightgrey">- 1</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 12 - 1 = 2x + 1</u> | <u style="color:lightgrey;background:lightgrey">- 1</u> |
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">10 = 2x</u> | <u style="color:lightgrey;background:lightgrey">: 2</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">10 = 2x</u> | <u style="color:lightgrey;background:lightgrey">: 2</u> |
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 5</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 5</u> | ||
| Zeile 74: | Zeile 94: | ||
::<math> \ 12 - 12 : 12 = 2x + 2 : 2 </math> | ::<math> \ 12 - 12 : 12 = 2x + 2 : 2 </math> | ||
| − | :: <math>\Leftrightarrow 12 - 1 = 2x + 1 | - 1 </math> | + | :: <math>\Leftrightarrow 12 - 1 = 2x + 1 \;\;\;\;\;\;\;\;| - 1 </math> |
| − | ::<math>\Leftrightarrow 10 = 2x | : 2 </math> | + | ::<math>\Leftrightarrow 10 = 2x \;\;\;\;\;\;\;\;| : 2 </math> |
| − | ::<math>\Leftrightarrow x = | + | ::<math>\Leftrightarrow x = 5 </math> |
::<math>\Rightarrow\mathbb{L}= \mathcal{f}5\mathcal{g}</math> | ::<math>\Rightarrow\mathbb{L}= \mathcal{f}5\mathcal{g}</math> | ||
| Zeile 84: | Zeile 104: | ||
| − | * < | + | * 2<sup>3</sup> + 3<sup>2</sup> = 5x + 17 |
<div align="right"><math>\mathbb{G}=\mathbb{N}</math></div> | <div align="right"><math>\mathbb{G}=\mathbb{N}</math></div> | ||
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 8 + 9 = 5x + 17 </u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 8 + 9 = 5x + 17 </u> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">17 = 5x + 17</u> |<u style="color:lightgrey;background:lightgrey"> - 17 </u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">17 = 5x + 17</u> | <u style="color:lightgrey;background:lightgrey"> - 17 </u> |
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">0 = 5x</u> | <u style="color:lightgrey;background:lightgrey">: 5 </u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">0 = 5x</u> | <u style="color:lightgrey;background:lightgrey">: 5 </u> |
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 0 ... wirklich? </u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = 0 ... wirklich? </u> | ||
| Zeile 102: | Zeile 122: | ||
:: <math>\Leftrightarrow 8 + 9 = 5x + 17 </math> | :: <math>\Leftrightarrow 8 + 9 = 5x + 17 </math> | ||
| − | ::<math>\Leftrightarrow 17 = 5x + 17 | - 17 </math> | + | ::<math>\Leftrightarrow 17 = 5x + 17 \;\;\;\;\;\;\;\;| - 17 </math> |
| − | ::<math>\Leftrightarrow 0 = 5x | : 5 </math> | + | ::<math>\Leftrightarrow 0 = 5x \;\;\;\;\;\;\;\;| : 5 </math> |
:: <math> Da \mathcal{f}0\mathcal{g} \notin \mathbb{N}\Rightarrow\mathbb{L}=\varnothing</math> | :: <math> Da \mathcal{f}0\mathcal{g} \notin \mathbb{N}\Rightarrow\mathbb{L}=\varnothing</math> | ||
| Zeile 115: | Zeile 135: | ||
<div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | <div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 9x + 5 = -13</u> | <u style="color:lightgrey;background:lightgrey">- 5</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> 9x + 5 = -13</u> | <u style="color:lightgrey;background:lightgrey">- 5</u> |
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">9x = -18</u> | <u style="color:lightgrey;background:lightgrey">: 9</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">9x = -18</u> | <u style="color:lightgrey;background:lightgrey">: 9</u> |
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = -2</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = -2</u> | ||
| Zeile 126: | Zeile 146: | ||
::<math> \ 3x + 8 + 6x - 3 = -13 </math> | ::<math> \ 3x + 8 + 6x - 3 = -13 </math> | ||
| − | :: <math>\Leftrightarrow 9x + 5 = -13 | - 5 </math> | + | :: <math>\Leftrightarrow 9x + 5 = -13 \;\;\;\;\;\;\;\;| - 5 </math> |
| − | ::<math>\Leftrightarrow 9x = -18| : 9 </math> | + | ::<math>\Leftrightarrow 9x = -18\;\;\;\;\;\;\;\;| : 9 </math> |
::<math>\Leftrightarrow x = -2 </math> | ::<math>\Leftrightarrow x = -2 </math> | ||
| Zeile 135: | Zeile 155: | ||
}} | }} | ||
| − | * <math> | + | * -3 <math>\cdot</math>(3 - 2x) + 2x = -21 |
<div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | <div align="right"><math>\mathbb{G}=\mathbb{Q}</math></div> | ||
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> -9 + 6x + 2x = -21</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> -9 + 6x + 2x = -21</u> | ||
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> -9 + 8x = -21</u> | <u style="color:lightgrey;background:lightgrey"> + 9</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey"> -9 + 8x = -21</u> | <u style="color:lightgrey;background:lightgrey"> + 9</u> |
| − | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">8x = -12</u> | <u style="color:lightgrey;background:lightgrey">: 8</u> | + | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">8x = -12</u> | <u style="color:lightgrey;background:lightgrey">: 8</u> |
:<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = -1,5</u> | :<math>\Leftrightarrow</math><u style="color:lightgrey;background:lightgrey">x = -1,5</u> | ||
| Zeile 153: | Zeile 173: | ||
:: <math>\Leftrightarrow -9 + 6x + 2x = -21</math> | :: <math>\Leftrightarrow -9 + 6x + 2x = -21</math> | ||
| − | ::<math>\Leftrightarrow -9 + 8x = -21| + 9 </math> | + | ::<math>\Leftrightarrow -9 + 8x = -21 \;\;\;\;\;\;\;\;| + 9 </math> |
| − | ::<math>\Leftrightarrow 8x = -12| : 8 </math> | + | ::<math>\Leftrightarrow 8x = -12 \;\;\;\;\;\;\;\;| : 8 </math> |
::<math>\Leftrightarrow x = -1,5 </math> | ::<math>\Leftrightarrow x = -1,5 </math> | ||
| Zeile 161: | Zeile 181: | ||
::<math>\Rightarrow\mathbb{L}= \mathcal{f}-1,5\mathcal{g}</math> | ::<math>\Rightarrow\mathbb{L}= \mathcal{f}-1,5\mathcal{g}</math> | ||
}} | }} | ||
| + | <br> | ||
| + | <br> | ||
| + | <br> | ||
| + | <div align="left">[[Aufgabentypen/Geometrie: Geometrische Ortlinien und Ortsbereiche |<math>\Rightarrow</math> Weiter zu 7. Klasse Geometrie]]</div> | ||
| + | <br> | ||
| + | <div align="left">[[Aufgabentypen/Geometrie: Achsenspiegelung|<math>\Leftarrow</math> Zurück zu 6. Klasse Geometrie]]</div> | ||
Aktuelle Version vom 17. Juli 2009, 13:17 Uhr
Gleichungen
|
Wie bei einer Waage müssen beide Seiten der Gleichung immer im Gleichgewicht sein. Dies erreichst du durch Äquivalenzumformungen, mit denen du beide Seiten der Gleichung einheitlich umstellst, ohne dass sich der Wert der Gleichung ändert. |
Deine neue Lieblings-Merkregel zum Umformen von Termen und Gleichungen:
Rechne immer Klammer vor Punkt vor Strich!
Wende diese Regel nun auf die folgenden Aufgaben an. Beachte immer die Grundmenge, die rechts steht.
Wenn du dir sicher bist, welche Äquivalenzumformung als nächstes kommt, darfst du durch Markieren des grauen Feldes den jeweils nächsten Schritt sichtbar machen!
Erst ganz am Ende kannst du dann dein Ergebnis durch Klick auf "Lösung anzeigen" überprüfen.
- Beispiel :
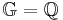
- 3x + 10 = 19
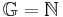
- 3x + 10 = 19 | - 10
 3x = 9 | : 3
3x = 9 | : 3
 x = 3
x = 3
- 9x - 18 = 27
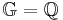
- 9x - 18 = 27 | + 18
 9x = 45 | : 9
9x = 45 | : 9
 x = 5
x = 5
- 12 - 12 : 12 = 2x + 2 : 2
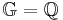
 12 - 1 = 2x + 1 | - 1
12 - 1 = 2x + 1 | - 1
 10 = 2x | : 2
10 = 2x | : 2
 x = 5
x = 5
- 23 + 32 = 5x + 17
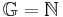
 8 + 9 = 5x + 17
8 + 9 = 5x + 17
 17 = 5x + 17 | - 17
17 = 5x + 17 | - 17
 0 = 5x | : 5
0 = 5x | : 5
 x = 0 ... wirklich?
x = 0 ... wirklich?
- 3x + 8 + 6x - 3 = -13
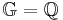
 9x + 5 = -13 | - 5
9x + 5 = -13 | - 5
 9x = -18 | : 9
9x = -18 | : 9
 x = -2
x = -2
- -3
 (3 - 2x) + 2x = -21
(3 - 2x) + 2x = -21
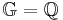
 -9 + 6x + 2x = -21
-9 + 6x + 2x = -21
 -9 + 8x = -21 | + 9
-9 + 8x = -21 | + 9
 8x = -12 | : 8
8x = -12 | : 8
 x = -1,5
x = -1,5

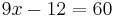
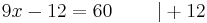
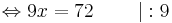
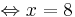

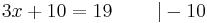
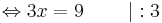
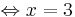

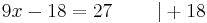
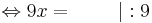
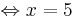

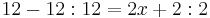
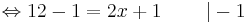
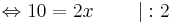
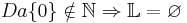
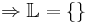
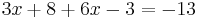
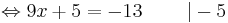
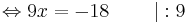
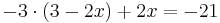
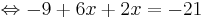
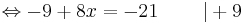

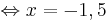
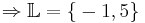
 Weiter zu 7. Klasse Geometrie
Weiter zu 7. Klasse Geometrie Zurück zu 6. Klasse Geometrie
Zurück zu 6. Klasse Geometrie
