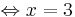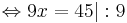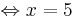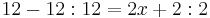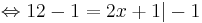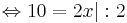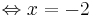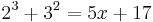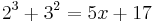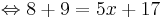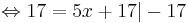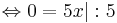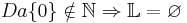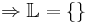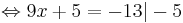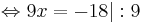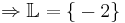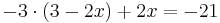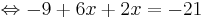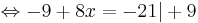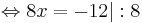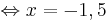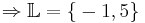7. Klasse:Algebra:Gleichungen 2
Aus DMUW-Wiki
Wende diese Regel nun auf die folgenden Aufgaben an. Beachte immer die Grundmenge, die rechts steht.
Wenn du dir sicher bist, welche Äquivalenzumformung als nächstes kommt, darfst du durch Markieren des grauen Feldes den jeweils nächsten Schritt sichtbar machen!
Erst ganz am Ende kannst du dann dein Ergebnis durch Klick auf "Lösung anzeigen" überprüfen.
- 3x + 10 = 19 | - 10
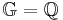
 3x = 9 | : 3
3x = 9 | : 3
 x = 3
x = 3
- 9x - 18 = 27 | + 18
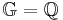
 9x = 45 | : 9
9x = 45 | : 9
 x = 5
x = 5
- 12 - 12 : 12 = 2x + 2 : 2
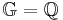
 12 - 1 = 2x + 1 | - 1
12 - 1 = 2x + 1 | - 1
 10 = 2x | : 2
10 = 2x | : 2
 x = 5
x = 5
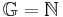
 8 + 9 = 5x + 17
8 + 9 = 5x + 17
 17 = 5x + 17 | - 17
17 = 5x + 17 | - 17
 0 = 5x | : 5
0 = 5x | : 5
 x = 0 ... wirklich?
x = 0 ... wirklich?
- 3x + 8 + 6x - 3 = -13
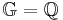
 9x + 5 = -13 | - 5
9x + 5 = -13 | - 5
 9x = -18 | : 9
9x = -18 | : 9
 x = -2
x = -2
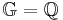
 -9 + 6x + 2x = -21
-9 + 6x + 2x = -21
 -9 + 8x = -21 | + 9
-9 + 8x = -21 | + 9
 8x = -12 | : 8
8x = -12 | : 8
 x = -1,5
x = -1,5