Lösungsvorschlag iv): Unterschied zwischen den Versionen
Aus DMUW-Wiki
K (Die Seite wurde neu angelegt: <div align="left"><math>\Leftarrow</math> Zurück</div>) |
K |
||
| (2 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| − | <div align="left">[[Zusammengesetzte Zufallsexperimente und Pfadregeln|<math>\Leftarrow</math> Zurück]]</div> | + | <big>möglicher Lösungsweg:</big> |
| + | |||
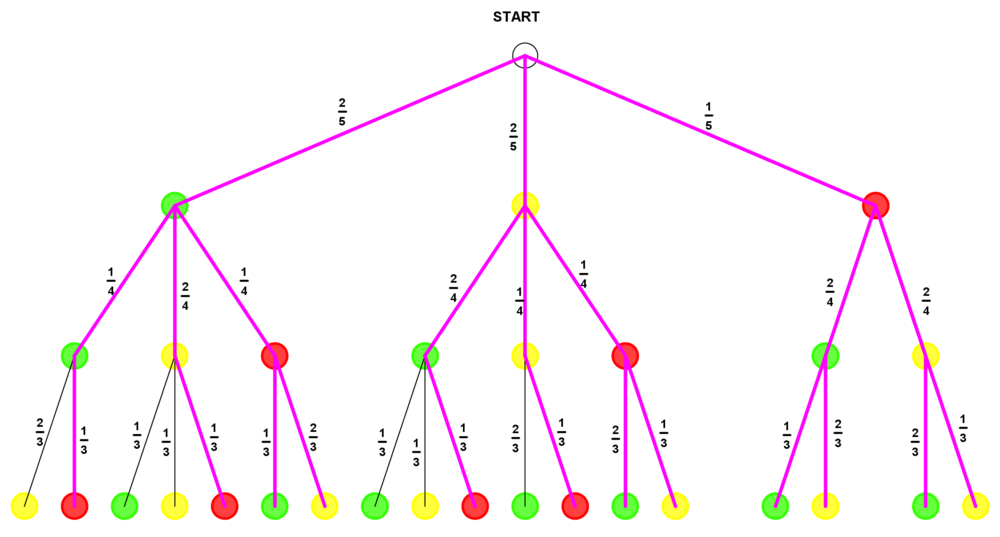
| + | Die Möglichkeiten, dass das rote Gummibärchen gezogen wird, sind im folgenden Baumdiagramm dargestellt: | ||
| + | |||
| + | [[Bild:Gummibärchendiagramm4.png|1000px]] | ||
| + | |||
| + | Berechnung der Wahrscheinlichkeit: | ||
| + | |||
| + | P(E<sub>4</sub>) = P({grün;grün;rot}) + P({grün;gelb;rot}) + P({grün;rot;grün}) + P({grün;rot;gelb}) + P({gelb;grün;rot}) + P({gelb;gelb;rot}) + P({gelb;rot;grün}) + P({gelb;rot;gelb}) + | ||
| + | |||
| + | + P({rot;grün;grün}) + P({rot;grün;gelb}) + P({rot;gelb;grün}) + P({rot;gelb;gelb}) = | ||
| + | |||
| + | |||
| + | = <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{2}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{2}{3}</math> + <math>\frac{2}{5}</math> <math>\cdot</math> <math>\frac{1}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + <math>\frac{1}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> + | ||
| + | |||
| + | |||
| + | + <math>\frac{1}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{2}{3}</math> + <math>\frac{1}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{2}{3}</math> + <math>\frac{1}{5}</math> <math>\cdot</math> <math>\frac{2}{4}</math> <math>\cdot</math> <math>\frac{1}{3}</math> = <math>\frac{3}{5}</math> = 60% | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | <div align="left">[[Zusammengesetzte Zufallsexperimente und Pfadregeln#Aufgabe 3|<math>\Leftarrow</math> Zurück]]</div> | ||
Aktuelle Version vom 28. September 2009, 18:17 Uhr
möglicher Lösungsweg:
Die Möglichkeiten, dass das rote Gummibärchen gezogen wird, sind im folgenden Baumdiagramm dargestellt:
Berechnung der Wahrscheinlichkeit:
P(E4) = P({grün;grün;rot}) + P({grün;gelb;rot}) + P({grün;rot;grün}) + P({grün;rot;gelb}) + P({gelb;grün;rot}) + P({gelb;gelb;rot}) + P({gelb;rot;grün}) + P({gelb;rot;gelb}) +
+ P({rot;grün;grün}) + P({rot;grün;gelb}) + P({rot;gelb;grün}) + P({rot;gelb;gelb}) =
= 



 +
+ 



 +
+ 



 +
+ 



 +
+ 



 +
+ 



 +
+ 



 +
+ 



 +
+ 



 +
+
+ 



 +
+ 



 +
+ 



 =
=  = 60%
= 60%
 Zurück
Zurück
